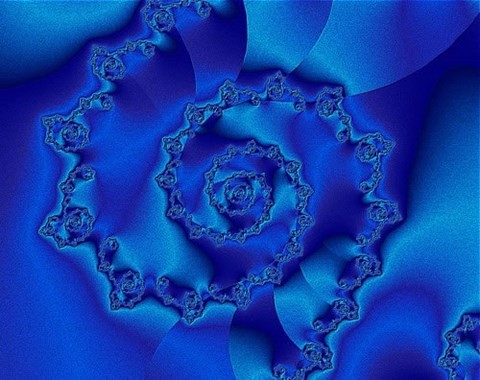
"The game of the chaos"
About the fractals
A fractal is a geometrical figure in which the same identical pattern is continuously repeated on a reduced scale. This means that enlarging the figure recurrent forms will be coming out, and at every enlargement it will reveal new details.
As opposed to every other geometrical figure, a fractal instead of loosing details while being enlarged, it acquires new particulars.A classical example of a fractal is represented by the sea coast: the coastal contours always show a very articulated system of capes and inlets, at any height and size you look at them. Other examples of fractals in nature are the rocks, the clouds, the galaxies, the notching of leaves, the branching out of the roots of a plant.
A brief history
The observation of reality through the fractal geometry dates back to the XIX century, but at the beginning the fractals were only considered as curious and bizarre objects or even "monstrous curves": so called by the scientific world of the age the curve discovered in 1890 by the mathematician Giuseppe Peano.
When the French mathematician of Polish origin, Benoit B. Maldelbrot at the beginning of the 60s, gave a strong development to the study of the matter; he wondered if the different and fascinating forms existing in nature replied only to the law of chaos, or if any nature morphology, although complex and articulated obeyed to some universal law.
The properties of the fractals

The properties of the fractals discovered by Mandelbrot were essentially two: the fractional dimension and the auto-resemblance otherwise called scale invariance.
The Euclidean geometry conventionally fixes a dimension to a straight line, two dimensions to a square, three dimensions to a cube; so it is sufficient to keep at a distance from the object in question so much it is necessary, that it is possible to define exactly its properties.
On the contrary, as regards the fractals, the dimension is fractional , whatever points of view and the adopted enlargement are considered, periodically they always present the same forms; and as each part is representative of the whole, it is auto-resemblance too.
One of the most curious properties of the fractals derives from this aspect: taken two points of the curve, even very close between them, the distance between them (measured along the curve) is always infinite. The area is finite but the perimeter is infinite.
To support his theories Mandelbrot produced some maths formulae relatively simple, that it seemed almost impossible that so simple algorithms could govern the apparently chaos of the creation.
Nowadays, thanks to computers, it is surely verified that matching these algorithms to recursive procedures (that repeat endlessly the geometries of the beginning) which can generate spatial symmetries of extreme complexity.
Art and fractals
But what is most amazing, from an artistic point of view, is that the so obtained imagines are of great beauty and equilibrium, pervaded with a chromatic harmony and of fluidity absolutely surprising and never by chance. Infact the fractal figures, even at a first look they seem abstract, in some way they respond to the same processes regulating the surrounding world and so they keep to our eyes a sort of familiarity suggesting us, in different ways and according to the individual sensibility, to a figurative interpretation too.
Michael Barnsley, another famous scholar of images produced by living organisms and of his turn father of "the diagram of Julia", today largely used for the creation of the fractals, who poetically defined the result of his studies as the "game of the chaos".
And if the artistic creation is generated, above all, from the passion and the desire to experiment, the play of the fractal art is well included in the research of new expressive formalities suggesting the reality but not directly representing it.
Here we are to the vision of fractal works presented on this site. For their creation, I have modified, with the computer, the different parameters of the starting point, making new and various forms; I have "sailed" inside them till to capture the points of maximum suggestion, and from these I have worked out pictorially and in a 3D the chosen _blankics, filtering them through my personal experience and taste, transforming them in small and I hope, true works of art.
Elio Stefano Pastore